Why π is in the normal distribution (beyond integral tricks)

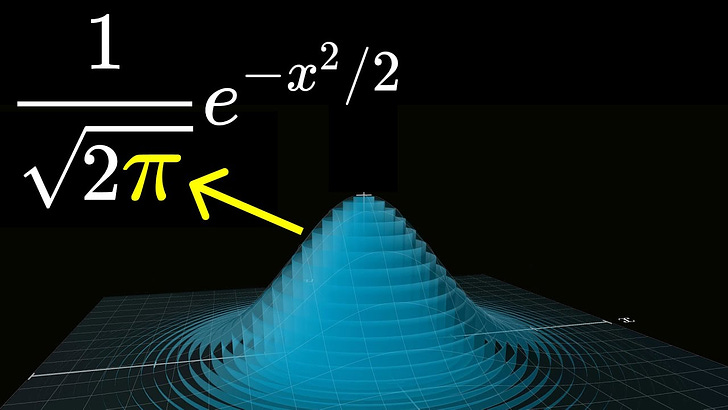
Here we look at classic proof explaining the pi in a Gaussian distribution, originally due to Poisson, which is just wildly fun to animate. On its own the proof feels out of the blue, so we also delve into the Herschel-Maxwell derivation for where a Gaussian comes from in the first place, and how it makes the famous proof much more reasonable.